
| The Concept of Time |
|---|

| The Concept of Time |
|---|
Home |
Summary: The concept of time and its correlation to entropy is discussed. We discuss time as a variable specific to the observer and his capability to make a PE1 measurement.  What is time? Can we understand it or should we just assume that it exists like everything else? What if nothing ever existed, would time still have a meaning? We will show that if a PE1 measurement can be made in as a single measurement, the entropy associated with the measurement will be zero and the concept of time has no significance. Furthermore if the number of measurements required to complete a PE1 measurement are finite, the entropy is still finite and time will of no essence. It is only when a PE1 measurement can not be completed, the time will become important and needs to be accounted for. We have introduced two different types of space, i-space and j-space. What will be the definition of time in i-space? We can not say. But we know a little about j-space and therefore let us try to develop a description of time from the information we have from the measurements made in j-space. Earlier when we described the progress of information in discrete measurement space or j-space, we introduced the concept of the <t=0j> state. The <t=0j> state represented the instant at which the measurements began in j-space. Consequently as more and more states were created with the progress of time the entropy increased. Thus an important assumption is made when the increment in time as measured by the observer in discrete space is considered equivalent to the increment in entropy. The observers have their own time-axis based on their capabilities. Therefore time is a part of the description from measurements. Then how do we correlate it to the universe as we know it? We can consider the universe itself as a PE1 measurement (in a Qbox if we may) which is yet to be completed.
 Thus we can simplify our description of time in terms of the applied measurement force in j-space. If the observer, for example Obsi, does not need to apply a measurement force, the time-axis does not start. The time in the discrete measurement space signifies the presence of an information source of much higher capability than that of the observer making the measurements. The time is taken as the characteristics of the discrete nature of the measurement space. The time has significance for Obsj-pair and ObsM both, however the space-time or (t, x, y, z) description, is specific to the macroscopic observer ObsM only. Before we close out this section we would like to discuss a rather interesting comment made by Kerson Huang and we quote, "The connection between quantum field theory and statistical mechanics rests on the apparent accident that the operator of time translation in quantum mechanics, exp (-itH/йд), where H is the Hamiltonian, maps into the density operator of the canonical ensemble exp (-βH), where β is the inverse temperature, if one makes the substitution t → iйдβ. That is, time corresponds to a pure-imaginary inverse temperature. The reason for this coincidence remains unknown. This mystery, and the deeper meaning of renormalization, are left for the reader's contemplation." Let us consider a situation in the discrete measurement space or j-space, where only a single PE1 state is created in the beginning, which is the initial <t = 0j> state represented by the q = 1 value. All the information from the δi (source for discrete space) is contained within this state. The temperature of such state will be measured by the discrete j-space observer as infinite. As the time progresses, more states are created and more and more information will be lost due to increasing entropy. As a consequence the measured temperature will go down. Therefore the time and measured temperature will have an inverse relationship in the description provide by the finite-capability observers in discrete j-space. We note the for the observer Obsi, there is only one shallow-well state referred to as <Si> state and hence entropy is zero and the time-axis will not exist. We can also say that once a PE1 measurement is completed the time-axis is terminated. Let us take a look at the Obsi criterion. The numbers with indices such as 0j, 1j,......., etc. represent the "measured" numbers in the discrete measurement space or j-space. We consider a field being measured in the j-space. Assume the it is characterized by its divergence and curl as, div F = 0 and curl F = 0. (i)
Per Obsi criterion we replace the zeros on the R.H.S. of the equation by measured numbers 0j as,
div F = 0j and curl F = 0j. (ii)
Therefore the field being measured as source-free and irrotational by ObsM in j-space, will always have a source and a boundary per Obsi who measures 0j as finite. These two important characteristics, i.e. the source and the boundary, are not necessarily with in the measurement resolution of the finite-capability observers of the discrete j-space. Consequently what is being measured by ObsM as zero, is actually indeterminate. The field F will be measured as with "content" by Obsi. But ObsM with its poor resolution may characterize it as non-existent whereas it is indeterminate at best. Thus if we had a situation expressed as, ∂bμ/∂xμ = 0, (iii)
we can apply the Obsi criterion to find that the field represented by the variable b if measured will be represented as, ∂bμ/∂xμ = 0j, (iv)
and it can not be source-free and it will have material characteristics which may not be visible in the low-energy measurement domain. If b can not be measured by ObsM then it is an indeterminate state rather than zero.
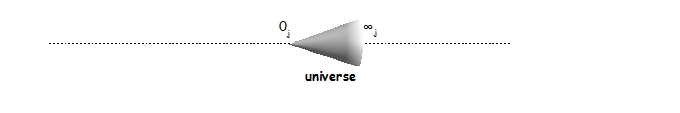
The limits of an infinite integral i.e. the interval [0, ∞] measured as an infinite interval by the discrete space observer, will be measured as a finite interval [0j, ∞j] by Obsi. Therefore effectively we can replace the lower and upper limits of an integral by the measured values of 0j and ∞j to obtain correct picture, valid within the observer's measurement domain. The whole discussion was started with the realization that zero can not be measured with an absolute precision. Similarly the infinitesimal period used for differentiation in j-space may contain multiple PE1 events measured by Obsi, which means that there will be stable structures below the measurement threshold of discrete space observers. Time in the discrete measurement space is either a temporary measure per Obsi (a shallow well with zero entropy) or an indeterminate entity per ObsM (A Q-box with infinite entropy). Added on 30th April 2014.
  Information on www.ijspace.org is licensed under a Creative Commons Attribution 4.0 International License.
|
What were the "boundary conditions" at the beginning of time? - Stephen Hawking, A Brief History of Time, Bantam Books. "The quantum mechanics does not, strictly speaking "know" the concept of the (discontinuous) "process" since all the temporal changes of the state take place continuously." - W. Pauli, General Principles of Quantum Mechanics, Springer-Verlag. Kerson Huang, Quantum Field Theory From Operators to Path Integrals, WILEY-VCH Verlag GmbH & Co. KGaA, Weinheim,2010. "Sensible mathematics involves neglecting a quantity when it is small - not neglecting it just because it is infinitely great and you do not want it!" - Dirac as quoted in Wikipedia. |